The Stack

I’m getting into the tougher parts of A Monster’s Expedition, and I’m noticing patterns. Islands are grouped into clusters, separated by long raft rides, each with its own distinct skin for the terrain and trees. And they function on the DROD model. That is, there don’t seem to be any new gameplay elements introduced after you start encountering double-length trees fairly early on, but each island cluster focuses on a particular interaction. You’ll have a group of puzzles where you need to position a log where it’ll stop another log from rolling too far, a group of puzzles where you have to push a log into the water in a place where it doesn’t make a complete bridge so you can climb onto it and push another log from an otherwise inaccessible direction, and so forth. Recognizing these themes can be a great help. Sometimes when I return to an incompletely-solved cluster, I re-solve some of the easier puzzles to remind myself what I should be looking for in the harder ones.

Another useful pattern: parity. When you push a single-length log lengthwise, it doesn’t shift, but rather, tumbles. If it’s positioned east-west, and you push it from the west side, it’ll pivot up and come to rest upright, one tile east of where it was. Once it’s upright, you can choose to push it down from the north or south, changing its direction. This scheme has the consequence that if you never roll a log from the side — and you frequently can’t roll logs without losing them over the edge of the island — then its possible positions and orientations are bound by parity in two directions. In any 2×2 square, you’ll have one tile where it can only be upright, one where it can only be east-west, one where it can only be north-south, and one where it can never be at all. Rolling the log, making it move until it hits an obstacle such as a rock or another log, can break this: if it rolls an odd number of spaces, its parity will be changed in the direction it rolled.

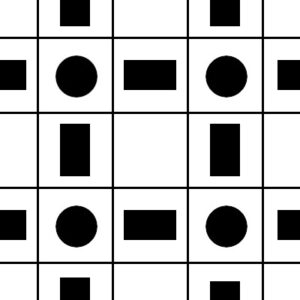Trees always start off upright, so I find it useful to think of the grid of the world in terms of places where a particular tree can be upright without rolling. This is a pattern you can just perceive, if you try. If you know where a log needs to go, and what orientation it needs to have there, then you can scan to see if it has the right parities, and if not, what obstacles could change that. It takes a good long time to reach the point in the game where it’s at all useful to think in these terms, though. Mostly you can just assume rocks are useful because they’re there.

A couple of levels on, and I’m once again finding myself spending a lot of my time clearing tar, not just because of those tar gates, but because clearing a room completely of tar has become a fairly common subject for Challenge scrolls. The mechanics of it are seeping into my dreaming mind, occupying my idle thoughts. Let me get some of this out in words.

Tar lies in multi-square puddles, which you can cut with your sword along any edge, except at the corners, which are vulnerable only to explosions — which is to say, invulnerable in rooms without bombs, which is most rooms. To remain stable, the puddles have to have a width of at least 2 in all places, both north-south and east-west; any square of tar that lacks a neighbor in either dimension will break off and start chasing you. Thus, the smallest stable configuration of tar is a simple 2×2 square. Since this puts all four tiles at a corner, such a square cannot be cleared. It’s the basic kernel of most unclearable tar shapes: if you can clear everything except a 2×2 square, you were doomed from the start. There are other invulnerable shapes, but they basically amount to multiple 2×2 squares stuck together by shared corners.

The basic clearable tar shape, on the other hand, is the 2×3 rectangle. Poke that in one of its vulnerable longer sides, and the remaining five squares break into monsters. This can be generalized. Given a 2xn strip, the only things you can do are cut off two rows at one end, or remove one row in the middle and split it into two pieces. If n is odd, you can cut off two rows repeatedly until it’s 2×3. If n is even, then it will still be even if you cut off two rows, and splitting it in the middle will always create one strip with an odd length and one with an even length; thus, you’re always going to wind up cutting it down to a 2×2 square at the end. So strips of this sort are solvable if their length is odd and unsolvable if their length is even. Many rooms have a checkerboard pattern on the floor, allowing you to tell odd from even at a glance.

Similar logic, which I’ll leave as an exercise for the reader, shows that a rectangle is solvable if and only if it has at least one odd side. It’s basically a matter of parity, an odd-or-even property that you can’t change with your sword, except with “odd” and “even” confusingly swapped: an odd length represents even parity and vice versa. That is, by assigning “even” to odd lengths, an nxm rectangle has even parity if either n or m has even parity, just as the product of two integers is even if either of them is even. It works out this way because of how splitting a rectangle into two pieces requires removing a row. When you split a rectangle in two, the pieces will have similar parity if the original had even parity, and opposite parity if the original was odd.

At any rate, all rectangles with even parity are solvable, but things get more complicated when we move beyond rectangles. You can have lumpy shapes with corners in inconvenient places that keep you from making the cuts you want. If you can reduce a shape to two separate 2×2 squares, it had even parity, but might still have been unsolvable. Odd parity is always unsolvable, though, no matter the shape. Assuming that Challenges are never completely impossible, it’s therefore safe to assume that the parity of any completely inert tar pool you’re supposed to clear will be even. But if there’s a Tar Mother in the room, making the tar expand at regular intervals, it’s possible for the parity to change. Thus, when you kill the Mother, it’s imperative to make sure that the remaining tar has the right parity if you intend to clear it all. I have no better way to do this for wiggly shapes than to attempt to clear it and see if it I wind up with a 2×2 square left over. But if I do, at least I know better than to keep trying from the same point.

Replaying the beginning of English Country Tune for comparison purposes seems to have turned into playing the whole game. I only got a bit more than halfway through it when I first played it, and, aided by memories, I’ve already gotten well past that point. In the process, I’ve re-encountered one of the most interesting puzzles I’ve ever seen. Let me describe it.

First, understand that the player avatar in this game is a square, which moves about on the surface of an agglomeration of blocks by flipping end over end. There’s a puzzle set where you’re coated with green paint that plants seeds on contact with special “garden” tiles, causing a sort of abstract bush or something to sprout when you leave the tile, rendering that square impassible. Your goal throughout this set is to paint a bush on every garden tile. In other words, it’s a series of puzzles about covering a set of squares without retracing your path, just like the red trap door puzzles in DROD. The three-dimensionality adds an extra twist or two, but nonetheless, I personally have found this sequence to be by far the easiest part of the game. It is, however, followed by a much trickier set, in which your flippy square has green paint on only one side, so that it alternates between consuming tiles and not consuming tiles. Among other things, this means that you have to take advantage of the corners of blocks to switch your parity.

That’s interesting, but the really interesting puzzle is the first one in the one-sided set. Instead of the normal puzzle interface, the game gives you a simple level editor, and challenges you to create a one-sided-paint puzzle out of nothing but blocks with garden tiles on every exposed face, and then solve it. This is actually pretty tricky to do. Most simple shapes cannot be covered completely with an alternating paintbrush. Presumably the author noticed this in the course of developing the puzzles, and realized that the level design problem he was solving was a pretty good puzzle in its own right, worthy of inclusion in the game. I don’t think I’ve seen this sort of level-design puzzle elsewhere, and it’s something I’d be interested in seeing more of.

I’ve gotten far enough into the game to see one more instance of a puzzle that uses the level editor, but it’s not the same: it asks you to create a shape that interacts with the rules in a particular way, but doesn’t ask you to make a solvable puzzle.